
Angular Momentum

Equipment
- Vernier rotary motion sensor with accessory kit
- Vertical support rod
- LabQuest 2
- Scale and ruler
In Part I we will measure the angular acceleration \( \alpha \) of a system under a known external torque taking in to account friction and will use out measurement to obtain the moment of inertia of the system. The equation which relates the net torque \( \tau_{net}\) to the moment of inertia and the angular acceleration of a rotating object is $$ \tau_{net} = I \alpha $$
We will measure the angular acceleration by measuring the angular velocity \( \omega \) as a function of time \( t\).
For a constant angular acceleration the equation which gives the dependence of \( \omega \) on t is $$ \omega = \omega_{0} + \alpha t $$
In Part II, conservation of angular momentum is investigated. The definition of angular momentum L is $$ L = I\omega $$
We will test whether angular momentum is conserved before and after the collision, using the relationship $$ I\omega = I′\omega′ $$
The dashed quantities refer to the angular momentum and angular velocity in the final state (after collision), and the undashed quantities refer to same variables in the initial state (before collision).
In order to increase the moment of inertia of the rotating object we will drop an aluminum disk onto the rotating platform, thus changing the moment of inertia, and measure the angular velocity \( \omega \) before and after the drop.
The apparatus used for this lab is sketched in the figure below. The Vernier rotary motion sensor is a bidirectional motion sensor that measures angular motion precisely and plots angular displacement, angular velocity and angular acceleration vs. time. This sensor is direction sensitive, meaning the signs of the vectors depend on the direction of the rotation of the sensor. For this experiment, we do not need to record the sign of each quantity, only the absolute value.

The motion sensor comes with a 3-‐step pulley (10mm, 29mm, 48mm diameter). A lightweight string can be wound around the pulleys; here we will only be using the largest one. When a small mass \(m\) is attached to the other end of the string, the tension \( T\) due to this small mass will create an external torque \(tau_{ext}\). The system is not frictionless. A frictional torque, \(tau_{fr}\), opposes the external torque resulting in a net torque,
$$ \tau_{net} = \tau_{ext} − \tau_{fr} $$The net torque gives the rotating platform an angular acceleration \( \alpha\) which is measured by the rate of increase of the angular velocity. The frictional torque \(\tau_{fr}\) causes a frictional deceleration \(\alpha_{fr}\) . The moment of inertia \(I\) of the rotating platform can be calculated from
$$ I = \frac{mr(g-r\alpha)}{\lvert\alpha_{fr}\rvert + \alpha} $$Where \( g \) is the acceleration due to gravity and \( r \) is the radius of the 3-‐step pulley around which you wind the string (\( r= 0.024\) m if you wind the string around the largest diameter). This equation can be derived by considering that the net torque is \( \tau_{net} = \tau_{ext} - \tau_{fr} \) the frictional torque is \( \tau_{fr} = I\alpha_{fr} \) and the applied torque is \( \tau_{ext} = Tr = mg − ar \).
Measurement of \( \alpha_{fr} \)
- 1. Connect the Rotary Motion Sensor to your data-‐collection interface and set the time to 20 seconds (by clicking in “Time” on the Meter screen).
- 2. Give the aluminum disk attached to the pulley a push so that it starts rotating slowly and then click “Collect” on the LabQuest2.
- 3. Go to the “Graph” screen, take the velocity vs. time graph and fit a straight line to your data by selecting the data on your graph and going to “Analyze” and then “Curve Fit”. Select “Linear“ in the fit equation option, and then click OK.
- 4. Record the value of the slope, which represents the angular acceleration α fr .
- 5. Repeat the measurement twice for a total of three measurements and consider the uncertainty in \( \alpha_{fr}\) to be half the difference between your maximum and minimum value of \( \alpha_{fr}\) .
Measurement of \( \alpha \)
- 1. Wind the string around the biggest diameter of the 3-‐step pulley and attach the small mass (10g) to the other end of the string. Make sure your string is long enough in order to collect enough data points when the weight of the mass \( m \) falls toward the floor.
- 2. Click "Collect Data" from LabQuest2 and release the system. Click stop when the mass has reached the lowest height.
- 3. Go to the “Graph” screen, take the velocity vs. time graph and fit a straight line to your data.
- 4. Record the value of the slope, which represents the angular acceleration \( \alpha \).
- 5. Repeat the measurement twice for a total of three measurements and consider the uncertainty in \( \alpha \) to be half the difference between your maximum and minimum value of \( \alpha \).
Testing of Conservation of Angular Momentum for Aluminum disks collision
Now, we will perform an experiment in which we change the initial moment of inertia by dropping an additional disk on top of the rotating platform. This drop (if done carefully) does not cause an external torque which would modify the initial angular momentum.
The final, or total, moment of inertia \( I′\) is equal to the sum of \( I\) (from previous section) and \(I_{disk}\) , the moment of inertia of the dropped disk.
Measurements for \( I_{disk} \)
- Measure the radius \( R \) of the disk with the ruler (approximately \( 24 \) mm) and use \(0.5\) mm for its absolute error.
- Measure the mass of the disk \( M\).
The Collision
- Remove the string from the 3-‐step pulley. Spin the rotating table. Press the “Collect” button, while the rotating table is spinning and drop the wide-‐hole aluminum disk.
- Go to the “Graph” screen and you should get an angular velocity vs. time graph that
looks roughly like this:
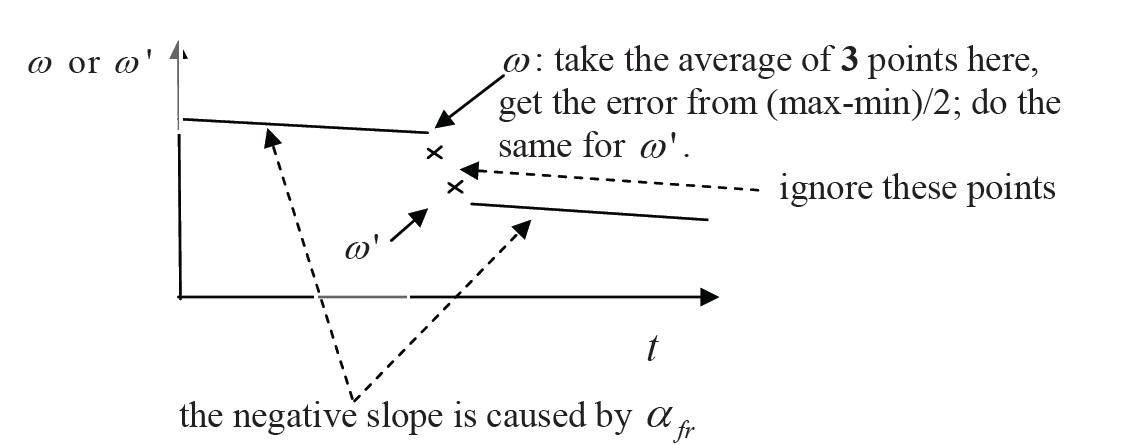
Note: The sign of the values for ω and ω′ (and the slope) may change depending on the direction the disk is spinning in. In general, you will expect to have a graph with a jump in the values of ω due to the dropped disk.
The higher line set of points corresponds to the initial angular velocity \( \omega \) and the lower line set of points corresponds to the final angular velocity \( \omegaʹ \). From the table of values record the angular velocity for 3 points on each side of the jump ω→ωʹ in your notebook (values close to the jump).
Calculate the average value of \( \omega \) and \( \omegaʹ \) and use half the difference between the maximum and minimum values of \( \omega \) and \( \omegaʹ \) as an estimate of the error in these two quantities.
Calculating I from \( \alpha \) and \( \alpha_{fr} \)
We have now measured everything we need to calculate the moment of inertia of the system using equation 5. The uncertainty in \(I\) will be mostly due to the uncertainties in your measured values of \( \alpha \) and \( \alpha_{fr} \) . The uncertainty in \( I \) can therefore be estimated using the formula: $$ \frac{\Delta I}{I} = \sqrt{ (\frac{\Delta\alpha}{\alpha})^2 + (\frac{\Delta\alpha_{fr}}{\alpha_{fr}})^2 } $$
Calculating \( I_{disk} \)
Next, calculate the moment of inertia of the disk \(I_{ disk} \) that is dropped from its mass \( M\) and its radius \(R\), using the formula. $$ I_{disk} = \frac{1}{2} MR^2 $$
Calculate the value of \( \Delta I_{ disk} \) taking in to account only the error in R : $$ \frac{\Delta I_{disk}}{I_{disk}} = 2 \frac{\Delta R}{R} $$
Calculating \( I' \)
The total moment of inertia \( Iʹ\) of the combined object (platform + dropped disk) is simply the sum of the two moments of inertia, $$ I' = I + I_{disk} $$
Record \( I' \) in your notebook. This value is only valid if the axis of rotation goes through both the center of the rotating table and the center of the dropped disk. That is, when you calculated \( I_{disk} \) you used an equation which was valid only if the axis of rotation went through its center) and the above equation is only complete if the disk is centered on teh platform.
Find the uncertainty in \( I′ \) by propagating the error of \( I \) and \(I_{disk}\): $$ \Delta I' = \sqrt{( ΔI )^2 + ( ΔI_{disk} )^2 } $$
Compare \(L\) and \(L′ \)
You are now ready to work out \(L\) and \(L′ \) from the definition, found in equation (1).
You should also calculate the error in \( L\) and \(Lʹ \) taking into account the uncertainties in the moments of inertia and the measured angular velocities. Once we have done this we can see if \(L\) and \(L′\) are the same within experimental uncertainty: $$ \frac{\Delta L}{L} = \sqrt{(\frac{\Delta I}{I})^2 + (2\frac{\Delta \omega}{\omega})^2} $$
Answer the following questions in your lab notebook:
- Was angular momentum conserved in your experiment (within experimental error)? If not, what are possible reasons? $$ L \stackrel{?}{=} L' $$
- Assume that you will test the conservation of angular momentum one more time, but now, you will keep the string and the hanging mass in the system. In other words, you wind the string around the pulley, with the mass on the other side and then, you will release the system. Before the mass reaches its lowest point you drop the other aluminum disk take the values of ω and ωʹ′ from the graph. Would you expect to observe conservation of angular momentum in this situation? Why or why not?
Hovering over these bubbles will make a footnote pop up. Gray footnotes are citations and links to outside references.
Blue footnotes are discussions of general physics material that would break up the flow of explanation to include directly. These can be important subtleties, advanced material, historical asides, hints for questions, etc.
Yellow footnotes are details about experimental procedure or analysis. These can be reminders about how to use equipment, explanations of how to get good results, or clarifications on details of frequent confusion.
Value is from NOAA for Latitude: 40.91597332879679, Longitude: -73.12491620370486, MSL Height: 41.0 Predicted gravity: 980269 +/- 2 milligals where a "gal" is a centimeter per second squared