The Geiger Counter and Radioactive Decay

Introduction

First, we will become familiar with the characteristics of the Geiger-Müller (GM) tube, including the properties of threshold, starting and operating voltages, GM plateau region and plateau slope. Then we will use the GM tube to perform a simple statistical experiment for the purpose of studying some of the techniques of statistical analysis. Finally, we will measure the half-life of an excited state of the $^{137}\mathrm{Ba}$ nucleus.

Apparatus

The basic GM circuit diagram is shown in Figure 1. The power supply and counter are one unit. The GM tube itself consists of a cylindrical outer shell which serves as a cathode and is grounded. In the center it has a coaxially mounted wire serving as anode. The cylinder is filled with an inert gas (typically Argon) at low pressure plus a small amount of “quenching” gas (e.g. ethyl alcohol).

A DC power supply provides a voltage across the tube in the range of a few hundred to over a thousand Volts depending on the requirements of the particular tube. Caution: The front window of the tube is very thin and delicate and should not be touched.

Radiation entering the tube can ionize some of the gas atoms, thereby freeing electrons which are accelerated towards the central wire by the electric field and gaining enough energy to cause further ionization. The result is a “cascade” or “avalanche” effect which produces a current pulse through the tube. This current pulse causes a voltage pulse across resistor $R$ , which is used to trigger a counting device that records the number of pulses. We are thus able to count the number of ionizing particles entering the tube. There is, however, no information about the initial particle energy. In the tube you are using a computer interface provides the DC power and plays the role of the oscilloscope to record counts. Open the Logger Pro program to access the counting software.

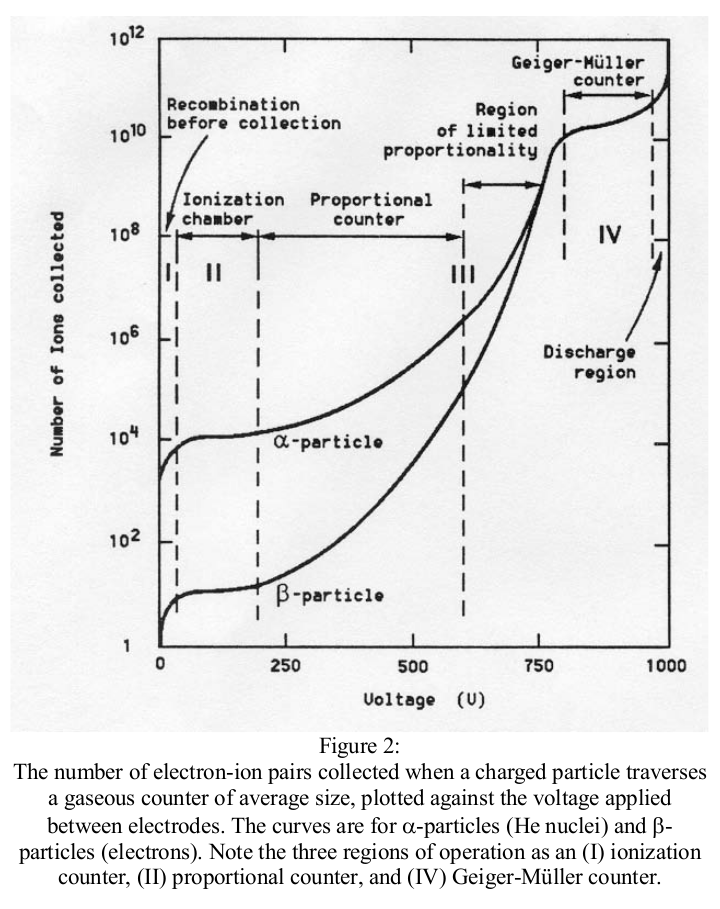
The purpose of the quenching additive to the gas is to effectively absorb UV-photons emitted from the electrodes when the ions produced in the multiplication process impact on the electrodes. Such photons may otherwise liberate secondary electrons (via the photo-electric effect) which may initiate the avalanche process all over again, thereby leading to catastrophic breakdown of the tube (i.e. a spark).

Familiarizing yourself with the Geiger Counter and statistical analysis

Measure the room background count rate. Record the number of counts $N$ per 10 sec interval for several intervals. Plot the number of counts per 10 sec interval as the abscissa and the number of times that number of counts occurred as the ordinate of a graph.
Determine the mean number of counts $\langle N \rangle$ and the standard deviation $\sigma$ . The result should approximate the “Poisson” distribution, for which the probability $P$ for observing $N$ counts in a given time interval is given by

$P(N)=\frac{\langle N \rangle^{N}e^{-\langle N \rangle}}{N!}\, , \, \sigma=\sqrt{\langle N \rangle}$

Compare your graph with this expression.

The decay of $^{137}Ba$

We will now measure the half-life of an excited state of the $^{137}Ba$ nucleus. Samples are prepared from a radioactive isotope of $^{137}Cs$ using a chemical that only dissolves Ba. You should ask your TA to prepare this sample for you. This isotope has too many neutrons to be stable; it decays by $\beta$ -decay into $^{137}Ba^{*}$ . This daughter nucleus is produced in an excited state, indicated by the asterisk ( $^{*}$ ). The excited state subsequently decays into the ground state of $^{137}Ba$ with a half-life of the excited state of 2.6 min. The decay of the excited state is detected by measuring the emitted $\gamma$ -rays of 0.66 MeV. This energy corresponds exactly to the energy difference between the excited state in $^{137}Ba^{*}$ and the ground state.

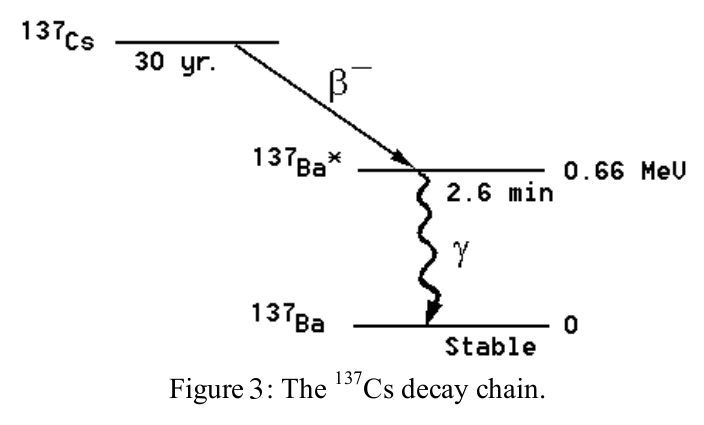
The $\gamma$ -ray emission rate $R(t)=-\frac{dN(t)}{dt}$ , where $N(t)$ is the number of $^{137}Ba^{*}$ nuclei present at time $t$ . The emission rate is proportional to $N(t)$ :

$\frac{dN(t)}{dt}=-\lambda N(t)$

where the proportionality factor $\lambda$ is defined as the decay constant. Integration of the above expression leads to

$N=N_{0}\exp(-\lambda t)$

where $N_{0}$ is the number of excited nuclei present at $t=0$ . The half-life $t_{1⁄2}$ is defined as the time it takes for the activity to be reduced by half; thus, at $t=t_{1⁄2}$ , $N=\frac{N_{0}}{2}$ . Derive the relationship between $\lambda$ and $t_{1⁄2}$ . Show that at any time $t+t_{1⁄2}$ , there will remain only one-half of the excited nuclei that were present at time $t$ !

Use a freshly prepared (!) sample of $^{137}Ba^{*}$ , place it under the Geiger counter, and measure the decay rate $\Delta N$ in some suitable time interval $\Delta t$ as a function of the elapsed time. Continue until the count rate is comparable with the background rate over the same time interval.
Correct your data for the background and plot the logarithm of the corrected count rate versus the time $t$ . You should obtain a straight line. Don't forget to put proper error bars on your data points. From the slope of the straight line you can obtain the half-life and the decay constant (including the errors). Compare with the accepted values.

Exploring beta/gamma radiation