Measurement of the electron charge

The purpose of this experiment is to measure the smallest unit into which electric charge can be divided, that is, the charge of an electron $e$. The method is the one proposed by R.A. Millikan in 1910. A small sphere of mass $m$ having a charge $q$ can be suspended in air by applying an electric field of field strength E to balance the gravitational force on it.

We then have

We neglect here the (very) small buoyant force. The charge $q$ will in general not be the electron charge but rather an integral multiple of it:

When the measurement is repeated several times, $e$ can be found as the largest common denominator of the measured charges $q$.

In the absence of an electric field, the electrons will reach a constant terminal velocity $v_T$ after a short time. The viscous force balances the gravitational force, so that the net force acting on the droplet is zero and we have:

where according to Stoke's law:

with $η$ the viscosity of air ($1.83×10^{-5} \mathrm{Nsm}^{-2}$ at $18^{\circ}\mathrm{C})$, $r$ the radius of the spheres ($\approx 0.50\mathrm{\mu m}$). From measuring the terminal velocity $v_{T}$ of free fall, the mass of the spheres can be determined.

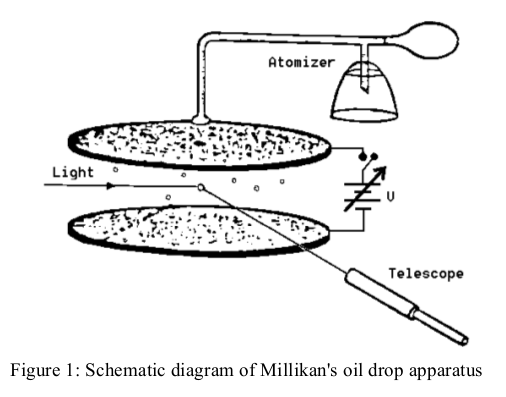
Figure 1 shows a schematic sketch of the experimental set-up. A closed chamber is placed between two capacitor plates 0.4 cm apart, in which a uniform electric field $E$ can be built up (remember, $E = V/d$). The chamber is illuminated by a small lamp. Charged spheres (a suspension of latex in water and alcohol) can be blown into the chamber through a tube and a nozzle, and be viewed there through a telescope with a calibrated scale (spacing of graduations 0.5 mm). Note that the telescope gives an inverted image.

1. Turn on the light and focus the telescope on the end of the nozzle which is used to blow spheres into the chamber. Then pull the nozzle back out of the field of view. Spheres can now be blown into the chamber by squeezing the rubber bulb.
2. Blow some spheres into the chamber and watch them (they will look tiny). They will quickly reach terminal velocity $v_{T}$ and should all fall at the same rate in the absence of an electric field. Measure this velocity by timing the travel of particles over a known distance with a stop watch. Repeat several times until you get a set of consistent values. Calculate the mass from the average terminal velocity. Compare to the value you obtain by calculating the mass using a density of $1.05 \mathrm{\,g\,cm^{-3}}$ (error estimate!).
3. Blow more spheres into the chamber and watch them falling. Now turn up the electric field. You will see them reverse direction and reach a new terminal velocity which now depends on their charge $q$. Since you want to measure the smallest charges, select one that is least affected by the $E$ field (i.e. one that is stationary at high fields) and adjust the voltage $V$ to hold it stationary. Write down the value of $V$ (hence $E$), and repeat the measurement 20 or more times trying to find spheres with slightly different charges.
4. Calculate for each measurement $q$, and determine from these values the charge unit e as the largest unit of charge consistent with your data. This is best done by guessing $n$ for each measurement (you should observe groupings in the distribution of the measured charges $q$ and assign an integer $n$ to every grouping) and plotting your measured values $q(n)$ versus $n$. The measurements should fall on a straight line with slope $e$. Compare with the literature value of $1.602×10^{-19}\mathrm{C}$,and discuss possible deviations.

The equipment we have doesn't always work very well. Therefore rather than follow the above procedure we propose you observe the phenomena using the actual setup and then take your data using a simulation: OPhysics Millikan Oil Drop http://www.teachscience.net/2011/02/07/millikan-oil-drop-simulation/

The simulation is very similar to the real experiment. Rather than produced many drops simultaneously you focus one drop at a time. To produce a drop click the button labelled “New Droplet”. You can change the rate at which time passes using the “Time Speed” slider. The terminal velocity can be measured using built in stop watch. The large divisions on the scale on the screen are cm (ie. the plates are about 2.5 cm apart). The stopping potential can be found by using the virtual “Power Supply”. Once you have determined the potential for one droplet, simply summon a new one and repeat until you have enough values.